basic performance characteristics of a system/block diagram of the measuring system/solid state voltmeter

STATIC CHARACTERISTICS :-

The static characteristics of an instrument are, in general, considered for instruments which are used to measure an unvarying process condition. All the static performance characteristics are obtained by one form or another of a process called calibration. There are a number of related definitions (or characteristics), which are described below, such as accuracy% precision, repeatability, resolution, errors, sensitivity, etc.

l. Instrument: A device or mechanism used to determine the present value of the quantity under measurement.

2. Measurement: The process of determining the amount, degree, or capacity by comparison (direct or

indirect) with the accepted standards of the system units being used.

3. Accuracy: The degree of exactness (closeness) of a measurement compared to the expected (desired)

value.

4. Resolution: The smallest change in a measured variable to which an instrument will respond.

5. Precision: A measure of the consistency or repeatability of measurements, i.e. successive readings

does not differ. (Precision is the consistency of the instrument output for a given value of input).

6. Expected value: The design value, i.e. the most probable value that calculations indicate one should

expect to measure.

7 Error: The deviation of the true value from the desired value.

8. Sensitivity: The ratio of the change in output (response) of the instrument to a change of input or measured variable.

DYNAMIC CHARACTERISTICS

Instruments rarely respond instantaneously to changes in the measured variables. Instead, they exhibit

slowness or sluggishness due to such things as mass, thermal capacitance, fluid capacitance or electric capacitance. In addition to this, pure delay in time is often encountered where the instrument waits for some reaction to take place. Such industrial instruments are nearly always used for measuring quantities

that fluctuate with time. Therefore, the dynamic and transient behavior of the instrument is as important as the static behavior.

The dynamic behavior of an instrument is determined by subjecting its primary element (sensing element) to some unknown and predetermined variations in the measured quantity. The three most

common variations in the measured quantity are as follows:

l. Step change in which the primary element is subjected to an instantaneous and finite change in

measured variable.

2. Linear change, in which the primary element is following a measured variable, changing linearly with

time.

3, Sinusoidal change, in which the primary element follows a measured variable, the magnitude of

which changes in accordance with a sinusoidal function of constant amplitude

.

The dynamic characteristics of an instrument are (i) speed of response,

(ii) Fidelity, (iii) lag, and (iv) dynamic error.

(i) Speed of Response: It is the rapidity with which an instrument responds to changes in the measured

quantity.

(ii) Fidelity: It is the degree to which an instrument indicates the changes in the measured variable

without dynamic error (faithful reproduction).

(iii) Lag: It is the retardation or delay in the response of an instrument to changes in the measured

variable.

(iv) Dynamic Error: It is the difference between the true values of a quantity changing with time and

the value indicated by the instrument, if no static error is assumed.

When measurement problems are concerned with rapidly varying quantities, the dynamic relations

between the instruments input and output are generally Defined by the use of differential equations

The method used to calculate the errors in an instrument:

ERROR IN MEASUREMENT

Measurement is the process of comparing an unknown quantity with an accepted

standard quantity. It involves connecting a measuring instrument into the system under consideration

and observing the resulting response on the instrument. The measurement thus obtained is a quantitative

measure of the so-called "true value" (since it is very difficult to define the true value, the term

"expected value" is used). Any measurement is affected by many variables; therefore the results rarely

reflect the expected value. For example, connecting a measuring instrument into the circuit under

consideration always disturbs (changes) the circuit, causing the measurement to differ from the expected

value. Some factors that affect the measurements are related to the measuring instruments themselves.

Other factors are related to the person using the instrument. The degree to which a measurement nears

the expected value is expressed in terms of the error of measurement. Error may be expressed either as

absolute or as percentage of error. Absolute error may be defined as the difference between the expected

value of the variable and the measured value of the variable, or

e = Y n - X n

Where e=absolute errors;

Yn=expected value;

Xn=measured value;

Therefore %error = (absolute value/expected value )*100=(e/Yn)*100

Therefore %error=

It is more frequently expressed as an accuracy rather than error.

Therefore A=1-

Where A is the relative accuracy

Accuracy is expressed as % accuracy

a=100%-%error

a=A*100% (where a=%accuracy)

 DC-Voltmeter:

A basic D'Arsonval movement can be converted into a dc voltmeter by adding a series

resistor known as multiplier, as shown in the figure. The function of the multiplier is to limit the

current through the movement so that the current does not exceed the full scale deflection value.

A dc voltmeter measures the potential difference between two points in a dc circuit or a circuit

component. To measure the potential difference between two points in a dc circuit or a circuit

component, a dc voltmeter is always connected

across them with the proper polarity. The value of

the multiplier required is calculated as follows.

Im: full scale deflection current of the movement

Rm : internal resistance of movement

Rs : Multiplier resistance

V: full range voltage of the instrument

From the circuit of Fig. 4.1

V= Im *( Rm+ Rs)

Rs = = -

therefore Rs = -

The multiplier limits the current through the movement, so as to not exceed the value of the full scale

deflection Ifsd.

The above equation is also used to further extend the

range in DC voltmeter'.

Multi range Voltmeter:

across the movement with a multi-position switch multi range ammeter, a number of shunts are connected

As in the case of an ammeter, to obtain a Similarly, a dc voltmeter can be converted into a multi range voltmeter by connecting a number of

resistors (multipliers) along with a range switch to provide a greater number of workable ranges. The below Figure shows a multi range

voltmeter using a three position switch and three multipliers R1, R2, and R3, for voltage values V1, V2, and V3. Fig 4.2 can be further modified to multipliers connected in series string, which is amore practical arrangement of the multiplier resistors of a multi range voltmeter. In this arrangement, the multipliers are connected in a series string, and the range selector selects the appropriate amount of resistance required in series with the movement.

This arrangement is advantageous compared to the previous one, because all multi1llier resistances

except the first have the standard resistance value and are also easily

available in precision tolerances. The first resistor or low range multiplier,

R4, is the only special resistor which has to be specially manufactured to meet the circuit requirements.

  

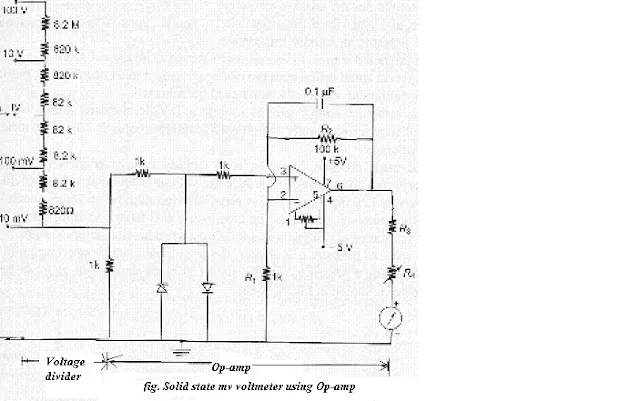
 Solid state voltmeter:

 

•  the working of solid state voltmeter:

 figure shows the circuit of an electronic voltmeter using an IC Op

Amp 741C.This is a directly coupled very high gain amplifier. The gain of the Op Amp can be adjusted to any suitable lower value by providing appropriate resistance between its output terminal, Pin No. 6, and inverting input, Pin No. 2, to provide a negative feedback. The ratio R2

/R1 determines the gain, i.e. 101 in this case, provided by the Op Amp. The 0.1 pF capacitor across the100 k resistance R is for stability under stray pick-ups Terminals 1 and 5 are called offset null terminals.

A 10 kΩ potentiometer is connected between these two offset null terminals with its centre tap connected to a - 5V supply. This potentiometer is called zero set and is used for adjusting zero output for zero input conditions.

The two diodes used are for IC protection. Under normal conditions, they are non-conducting, as the

maximum voltage across them is l0 mV. If an excessive voltage, say more than 100 mV appears across

them, then depending upon the polarity of the voltage, one of the diodes conducts and protects the IC. A

μA scale of 50 - 1000 μA full scale deflection can be used as an indicator. Ro is adjusted to get maximum full scale deflection.

Block diagram of the measuring system:

The generalized measuring system consists of three main functional elements. They are,

1. Primary sensing element, which senses the quantity under measurement.

2. Variable conversion element, which modifies suitably the output of the primary sensing element

3. Data presentation element that renders the indication on a calibrated scale.

1. Primary Sensing Element

The measurement first comes into contact with primary sensing element where the conversion takes

place. This is done by a transducer which converts the measurement (or) measured quantity into a usable

electrical output. The transduction may be from mechanical, electrical (or) optical to any related form.

2. Variable Conversion Element

The output of the primary sensing element is in the electrical form suitable for control, recording and

display. For, the instrument to perform the desired function, it may be necessary to convert this output to

some other suitable for preserving the original information. This function is performed by the variable

conversion element. A system may require one (or) more variable conversion suitable to it.

(a) Variable Manipulation Element

The signal gets manipulated here preserving the original nature of it. For example, an amplifier accepts a

small voltage signal as input and produces a voltage, of greater magnitude. The output is the same

voltage but of higher value, acting as a voltage amplifier. Here the voltage amplifier acts as a variable

manipulation element since it amplifies the voltage. The element that follows the primary sensing

element in a measurement system is called signal conditioning element. Here the variable conversion

element and variable manipulation element are collectively called as Data conditioning element (or)

signal conditioning element.

(b) Data Transmission Element

The transmission of data from one another is done by the data transmission element. In case of

spacecrafts, the control signals are sent from the control stations by using radio signals.

The stage that follows the signal conditioning element and data transmission element collectively is

called the intermediate stage.

(c).Data Presentation Element

The display (or) readout devices which display the required information about the measurement, forms

the data presentation element. Here the information of the measurand has to be conveyed for,

monitoring, Control (or) analysis purposes.

(a). 1t case of data to be monitored, visual display devices are needed like ammeters; voltmeters and so

on are used.

(b)In case of data to be recorded, recorders like magnetic tapes, T.V equipment, and storage type C.R T,

printers and so on are used.