Hay’s Bridge Circuit and the expression for the unknown element at balance.
Hay’s Bridge :
Circuit and derives the expression for the unknown element at balance,
The Hay Bridge, fig. 3.1 differs from Maxwell's bridge by having a resistance R1 in series with a standard capacitor C1 instead of a parallel. For large phase angles, R1 needs to be low; therefore, this bridge is more convenient for measuring high Q coils.
For Q=10, the error is ± 1 %, and for Q = 30, the is ±
0.1%. Hence Hay's bridge is preferred for coils with a
high Q, and Ma bridge for coils with a low Q.
At balance
Substiting these values in the balance equation we get
Equating the real and imaginary terms we have
Solving for Lx and Rx we have
Substituting for Rx in equation 1.1
Multiply both sides by C1 we can get,
Therefore,
Substituing for Lx in eq 1.2 we get
\
The term ω appears in the expression for both LX and RX. This indicates that the bridge is frequency
sensitive.
Hay Bridge is also used in the measurement of incremental inductance. The inductance balance
equation depends on the losses of the inductor (or Q) and also on the operating frequency.
AN inconvenient feature of this bridge is that the equation giving the balance condition for
inductance, contains the multiplier 1/ (1 + 1/Q2).
The inductance balance thus depends on its Q and frequency.
For a value of Q greater than 10, the term L/Q2 will be smaller than 1/100 and can be therefore neglected.
Therefore LX = R2 R3 C1 which is the same as Maxwell's equation. But for inductors with a Q less than
10, the 1/Q2 term cannot be neglected. Hence this bridge is not suited for measurements of coils having
Q less than 10. A commercial bridge measure from 1μH - 100 H with ± 2% error.










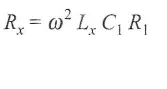



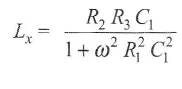




i need a comprehensive note on hartshorn bridge used for measuring mutual inductance
ReplyDeletepls now, pls and pls
Thanx sir....
ReplyDeletethnx sir... :)
ReplyDeletethank you :)
ReplyDeletetnk u sir. more grace
ReplyDelete